Mathematical tools: Difference between revisions
Jump to navigation
Jump to search
Wiki-cours (talk | contribs) No edit summary |
Wiki-cours (talk | contribs) No edit summary |
||
| Line 19: | Line 19: | ||
* 30/11 Green's function | * 30/11 Green's function | ||
* 07/12 Orthogonal polynomials | * 07/12 Orthogonal polynomials | ||
* | * 10/01 '''Final Exam (3h)''' on everything | ||
'''incomplete bibliography''' : | '''incomplete bibliography''' : | ||
* [http://physics.bgu.ac.il/~gedalin/Teaching/Mater/mmp.pdf Mathematical Methods for Physicists] -- T. L. Chow '''(free pdf)'''. | * [http://physics.bgu.ac.il/~gedalin/Teaching/Mater/mmp.pdf Mathematical Methods for Physicists] -- T. L. Chow '''(free pdf)'''. | ||
* [https://www.elsevier.com/books/mathematical-methods-for-physicists/arfken/978-0-12-384654-9 MATHEMATICAL METHODS FOR PHYSICISTS], George B. Arfken & H. J. Weber | * [https://www.elsevier.com/books/mathematical-methods-for-physicists/arfken/978-0-12-384654-9 MATHEMATICAL METHODS FOR PHYSICISTS], George B. Arfken & H. J. Weber | ||
* [http://www.goldbart.gatech.edu/PostScript/MS_PG_book/bookmaster.pdf Mathematics for Physics] -- M. Stone & P. Goldbart '''(free pdf)''' | * [http://www.goldbart.gatech.edu/PostScript/MS_PG_book/bookmaster.pdf Mathematics for Physics] -- M. Stone & P. Goldbart '''(free pdf)''' | ||
* [https://press.princeton.edu/titles/10773.html Group Theory in a Nutshell for Physicists] -- Anthony Zee. | * [http://www.worldscientific.com/worldscibooks/10.1142/9733 Physics and Mathematical Tools: Methods and Examples] -- A. Alastuey, M. Clusel, M. Magro, P. Pujol. | ||
* [https://press.princeton.edu/titles/10773.html Group Theory in a Nutshell for Physicists] -- Anthony Zee. (if you want to learn group theory) | |||
'''Evaluation''' (3 ECTS) | '''Evaluation''' (3 ECTS) | ||
* continuous assessment | * continuous assessment | ||
* final exam : 3 hours, written exam | * final exam : 3 hours, written exam | ||
Revision as of 11:07, 22 November 2017

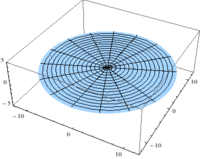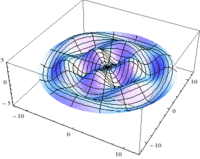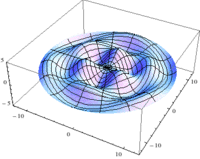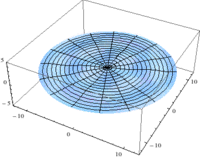
Bessel function for the drums
Lecturer : Guillame Roux
Syllabus: Course built on miscellaneous small chapters, based on examples. The goal is to recall and/or introduce useful mathematical tools with hands on.
Approximate Schedule for the 13 courses:
- 07/09 Functionals derivatives I -- tutorial
- 14/09 Functionals derivatives II
- 21/09 Lagrange multipliers and functional integration
- 28/09 Complex analysis + 30min test on 1.2.3 -- tutorial
- 05/10 Fourier transform -- tutorial
- 12/10 Principal value -- tutorial
- 19/10 Kramers-Krönig relations
- 26/10 Gaussian integrals + 60min test on 4.5.6.7-- tutorial
- 02/11 Wick's theorem
- 16/11 Saddle points methods
- 23/11 Linear algebra
- 30/11 Green's function
- 07/12 Orthogonal polynomials
- 10/01 Final Exam (3h) on everything
incomplete bibliography :
- Mathematical Methods for Physicists -- T. L. Chow (free pdf).
- MATHEMATICAL METHODS FOR PHYSICISTS, George B. Arfken & H. J. Weber
- Mathematics for Physics -- M. Stone & P. Goldbart (free pdf)
- Physics and Mathematical Tools: Methods and Examples -- A. Alastuey, M. Clusel, M. Magro, P. Pujol.
- Group Theory in a Nutshell for Physicists -- Anthony Zee. (if you want to learn group theory)
Evaluation (3 ECTS)
- continuous assessment
- final exam : 3 hours, written exam