Mathematical tools: Difference between revisions
Jump to navigation
Jump to search
Wiki-cours (talk | contribs) mNo edit summary |
Wiki-cours (talk | contribs) mNo edit summary |
||
| Line 17: | Line 17: | ||
* 30/09 Fourier transform -- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/TDFourier.pdf notes & tutorial] <!-- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanFourier.pdf correction] --> | * 30/09 Fourier transform -- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/TDFourier.pdf notes & tutorial] <!-- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanFourier.pdf correction] --> | ||
* 07/10 Principal value, Kramers-Krönig relations -- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/TDkramers.pdf tutorial] <!-- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanTDkramers.pdf correction] --> | * 07/10 Principal value, Kramers-Krönig relations -- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/TDkramers.pdf tutorial] <!-- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanTDkramers.pdf correction] --> | ||
* 21/10 Gaussian integrals and Wick's theorem [http://lptms.u-psud.fr/membres/groux/enseignements/Math/GaussianAsymptotics.pdf notes & tutorial] -- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/TDsteepest.pdf tutorial] '''+test (3h)''' => 17:30 <!-- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanTDgaussiennes.pdf correction] --> | * 21/10 Gaussian integrals and Wick's theorem [http://lptms.u-psud.fr/membres/groux/enseignements/Math/GaussianAsymptotics.pdf notes & tutorial] -- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/TDsteepest.pdf tutorial] '''+ 1h test (total 3h)''' => 17:30 <!-- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanTDgaussiennes.pdf correction] --> | ||
* 25/11 Saddle points methods '''(3h)''' => 17:30 <!-- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanLaplaceMethod.pdf Laplace method] -- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanTDsteepest.pdf correction] --> | * 25/11 Saddle points methods '''(3h)''' => 17:30 <!-- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanLaplaceMethod.pdf Laplace method] -- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanTDsteepest.pdf correction] --> | ||
* 02/12 Linear algebra -- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/TDlinearAlgebra.pdf tutorial] '''(3h)''' => 17:30 <!-- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanTDLinearAlgebra.pdf correction] --> | * 02/12 Linear algebra -- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/TDlinearAlgebra.pdf tutorial] '''(3h)''' => 17:30 <!-- [http://lptms.u-psud.fr/membres/groux/enseignements/Math/scanTDLinearAlgebra.pdf correction] --> | ||
Revision as of 13:37, 3 September 2021

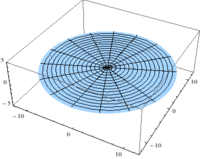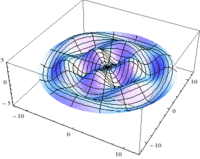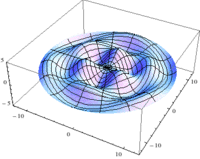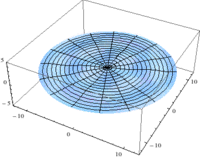
Bessel function for the drums
Master : an option of the Master 2 Physics of complex system
Lecturer : Guillame Roux
Syllabus: Course built on miscellaneous small chapters, based on examples. The goal is to recall and/or introduce useful mathematical tools with hands on.
Location: University Paris-Saclay, hbar 625 building, amphi A3, mostly 14:30--16:30,see website
2021-2022: Approximate Schedule for the 13 courses:
- 09/09 Functionals derivatives I -- tutorial (beware first lecture @11:15 at the slot of biophysics lecture!!)
- 09/09 Functionals derivatives II
- 16/09 Continuous groups and Lie algebra -- notes & tutorial
- 23/09 Complex analysis -- notes & tutorial
- 30/09 Fourier transform -- notes & tutorial
- 07/10 Principal value, Kramers-Krönig relations -- tutorial
- 21/10 Gaussian integrals and Wick's theorem notes & tutorial -- tutorial + 1h test (total 3h) => 17:30
- 25/11 Saddle points methods (3h) => 17:30
- 02/12 Linear algebra -- tutorial (3h) => 17:30
- 09/12 Green's function: static case -- tutorial
- 16/12 Green's function: causality and propagation
[?? * 17/12 Orthogonal polynomials and Special functions -- notes & tutorial ??
- Week of 20/01 Final Exam (3h) 9:00-12:00, on everything
incomplete bibliography :
- Mathematical Methods for Physicists -- T. L. Chow (free pdf).
- MATHEMATICAL METHODS FOR PHYSICISTS, George B. Arfken & H. J. Weber
- Mathematics for Physics -- M. Stone & P. Goldbart (free pdf)
- Mathematical physics: a modern introduction to its foundations -- S. Hassani
- Mathematical Methods: For Students of Physics and Related Fields -- S. Hassani
- Physics and Mathematical Tools: Methods and Examples -- A. Alastuey, M. Clusel, M. Magro, P. Pujol.
- Group Theory in a Nutshell for Physicists -- Anthony Zee. (if you want to learn group theory)
Evaluation (3 ECTS)
- continuous assessment (1/3 of the final grade): each assessment is graded over 10, this makes a grade over 20. The average grade of the class is typically 14/20.
- final exam : 3 hours, written exam (2/3 of the final grade)
- you are allowed to bring your notes and the distributed documents at the tests and exam, but nothing else.
Previous test
- 2017--2018 : Test on complex calculus -- correction (some typos in modulus squares in the correction...)
- 2018--2019 : Test on complex calculus -- correction
- 2019--2020 : Test on complex calculus -- correction
Previous final exams
- 2017--2018 : subject -- correction
- 2018--2019 : subject -- correction
- 2019--2020 : subject -- correction
- 2020--2021 : subject