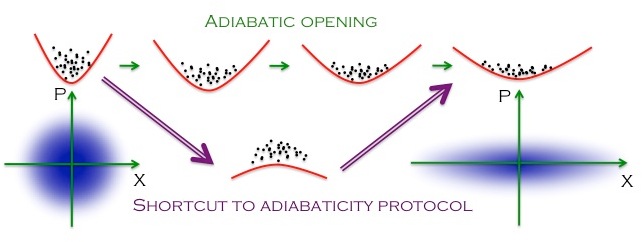
In 1872, Boltzmann established a key bridge between microscopic dynamics and macroscopic irreversibility, through the H theorem: a dilute gas thereby evolves towards equilibrium, where it is ruled by Maxwell-Boltzmann statistics. The Austrian physicist soon after realized that when the gas is confined by a harmonic trap, more general such statistics could exist, where the mean size and temperature of the gas oscillate with time, without dissipation. A Franco-Spanish collaboration has shown that this class of many-body solutions could be extended, in particular to time-dependent trapping. These new exact solutions for the Boltzmann equation hold for arbitrary short-range interaction potentials, and can be used to propose an original molecular manipulation technique. The idea, in a reverse engineering perspective, is to work out what time-dependent harmonic confining potential is required to achieve a fast prescribed time evolution of the system’s state. It then becomes possible to transform a gas from an equilibrium state to another one in an arbitrarily small time span. This shortcuts the traditional ‘adiabatic’ technique, which realizes the same goal, but in an unacceptably large duration. Achieving fast cooling of an assembly of particles is desirable in a variety of contexts, in particular to devise accurate atomic clocks.