|
|
| (252 intermediate revisions by the same user not shown) |
| Line 1: |
Line 1: |
| In this set of problems, we use the replica method to study the equilibrium properties of a prototypical toy model of glasses, the spherical <math>p</math>-spin model.
| | <strong>Goal: </strong> deriving the equilibrium phase diagram of the Random Energy Model (REM). Notion such as: freezing transition, entropy crisis, condensation, overlap distribution. <br> |
| | | <strong> Techniques: </strong> saddle point approximation, thermodynamics, probability theory. |
| | | <br> |
| | |
| === Problem 1: the energy landscape of the REM ===
| |
| | |
| In this exercise we study the number <math> \mathcal{N}(E)dE </math> of configurations having energy <math> E_\alpha \in [E, E+dE] </math>. This quantity is a random variable. For large <math> N </math>, we will show that its <em> typical value </em> scales as
| |
|
| |
|
|
| |
|
| | == Problems== |
| | === Problem 2: the REM: freezing transition, condensation & glassiness === |
| | In this Problem we compute the equilibrium phase diagram of the model, and in particular the quenched free energy density <math>f(\beta):=f_\infty(\beta) </math> which controls the scaling of the typical value of the partition function, <math>Z_N(\beta) \sim e^{-N \beta \, f(\beta) +o(N) } </math>. We show that the free energy equals to |
| <center><math> | | <center><math> |
| \mathcal{N}(E) = e^{N \Sigma\left( \frac{E}{N}\right) + o(N)}, \quad \quad \Sigma(\epsilon)
| | f(\beta) = |
| = | |
| \begin{cases} | | \begin{cases} |
| \log 2- \epsilon^2 \quad &\text{ if } |\epsilon| \leq \sqrt{\log 2} \\
| | &- \left( T \log 2 + \frac{1}{2 T}\right) \quad \text{if} \quad T \geq T_f\\ |
| 0 \quad &\text{ if } |\epsilon| >\sqrt{\log 2}
| | & - \sqrt{2 \,\log 2} \quad \text{if} \quad T <T_f |
| \end{cases}
| | \end{cases} \quad \quad T_f= \frac{1}{ \sqrt{2 \, \log 2}}. |
| </math></center> | | </math></center> |
| | At <math> T_f </math> a transition occurs, often called freezing transition: in the whole low-temperature phase, the free-energy is “frozen” at the value that it has at the critical temperature <math>T= T_f </math>. <br> |
| | We also characterize the overlap distribution of the model: the overlap between two configurations <math> \vec{\sigma}^\alpha, \vec{\sigma}^\beta </math> is |
| | <math>q_{\alpha \, \beta}= N^{-1} \sum_{i=1}^N \sigma_i^\alpha \, \sigma_i^\beta</math>, and its distribution is |
| | <center> |
| | <math> |
| | P_{N, \beta}(q)= \sum_{\alpha, \beta} \frac{e^{-\beta E(\vec{\sigma}^\alpha)}}{Z_N}\frac{e^{-\beta E(\vec{\sigma}^\beta)}}{Z_N}\delta(q-q_{\alpha \beta}). |
| | </math> |
| | </center> |
|
| |
|
| The function <math> \Sigma(\epsilon) </math> is the entropy of the model, and it is sketched in Fig. X. The point where the entropy vanishes, <math> \epsilon=- \sqrt{\log 2} </math>, is the energy density of the ground state, consistently with what we obtained in the lecture. The entropy is maximal at <math> \epsilon=0 </math>: the highest number of configurations have vanishing energy density.
| |
|
| |
|
| |
| <ol>
| |
| <li> <em> Averages: the annealed entropy.</em> We begin by computing the “annealed" entropy <math> \Sigma^A </math>, which is the function that controls the behaviour of the average number of configurations at a given energy, <math> \overline{\mathcal{N}(E)}= \text{exp}\left(N \Sigma^A\left( \frac{E}{N} \right)+ o(N)\right) </math>. Compute this function using the representation <math> \mathcal{N}(E)dE= \sum_{\alpha=1}^{2^N} \chi_\alpha(E) dE \;</math> [with <math> \chi_\alpha(E)=1</math> if <math> E_\alpha \in [E, E+dE]</math> and <math> \chi_\alpha(E)=0</math> otherwise], together with the distribution <math> p(E)</math> of the energies of the REM configurations. When does <math> \Sigma^A </math> coincide with the entropy defined above (which we define as the “quenched” entropy in the following)?</li>
| |
| </ol>
| |
| <br>
| |
|
| |
| <ol start="2">
| |
| <li><em> Self-averaging.</em> For <math> |\epsilon| \leq \sqrt{\log 2} </math> the quantity <math> \mathcal{N} </math> is self-averaging: its distribution concentrates around the average value <math> \overline{\mathcal{N}} </math> when <math> N \to \infty </math>. Show that this is the case by computing the second moment <math> \overline{\mathcal{N}^2} </math> and using the central limit theorem. Show that this is no longer true in the region where the annealed entropy is negative: why does one expect fluctuations to be relevant in this region?</li>
| |
| </ol>
| |
| <br>
| |
|
| |
|
| <ol start="3">
| |
| <li> <em> Rare events vs typical values.</em> For <math> |\epsilon| > \sqrt{\log 2} </math> the annealed entropy is negative: the average number of configurations with those energy densities is exponentially small in <math> N </math>. This implies that the probability to get configurations with those energy is exponentially small in <math> N </math>: these configurations are rare. Do you have an idea of how to show this, using the expression for <math> \overline{\mathcal{N}}</math>? What is the typical value of <math> \mathcal{N} </math> in this region? Justify why the point where the entropy vanishes coincides with the ground state energy of the model.</li>
| |
| </ol>
| |
|
| |
|
| |
| '''Comment:''' this analysis of the landscape suggests that in the large <math> N </math> limit, the fluctuations due to the randomness become relevant when one looks at the bottom of their energy landscape, close to the ground state energy density. We show below that this intuition is correct, and corresponds to the fact that the partition function <math> Z </math> has an interesting behaviour at low temperature.
| |
|
| |
| === Problem 2: the free energy and the freezing transition ===
| |
|
| |
| We now compute the equilibrium phase diagram of the model, and in particular the quenched free energy density <math>f </math> which controls the scaling of the typical value of the partition function, <math>Z \sim e^{-N \beta \, f +o(N) } </math>. We show that the free energy equals to
| |
| <center><math>
| |
| f =
| |
| \begin{cases}
| |
| &- \left( T \log 2 + \frac{1}{4 T}\right) \quad \text{if} \quad T \geq T_c\\
| |
| & - \sqrt{\log 2} \quad \text{if} \quad T <T_c
| |
| \end{cases} \quad \quad T_c= \frac{1}{2 \sqrt{\log 2}}.
| |
| </math></center>
| |
| At <math> T_c </math> a transition occurs, often called freezing transition: in the whole low-temperature phase, the free-energy is “frozen” at the value that it has at the critical temperature <math>T= T_c </math>.
| |
|
| |
|
| <ol> | | <ol> |
| <li><em> The thermodynamical transition and the freezing.</em> | | <li><em> The freezing transition.</em> |
| The partition function the REM reads | | The partition function the REM reads |
| <math> | | <math> |
| Z = \sum_{\alpha=1}^{2^N} e^{-\beta E_\alpha}= \int dE \, \mathcal{N}(E) e^{-\beta E}.
| | Z_N(\beta) = \sum_{\alpha=1}^{2^N} e^{-\beta E_\alpha}= \int dE \, \mathcal{N}_N(E) e^{-\beta E}. |
| </math> | | </math> |
| Using the behaviour of the typical value of <math> \mathcal{N} </math> determined in Problem 1, derive the free energy of the model (hint: perform a saddle point calculation). What is the order of this thermodynamic transition? | | Using the behaviour of the typical value of <math> \mathcal{N}_N </math> determined in Problem 1, derive the free energy of the model (hint: perform a saddle point calculation). What is the order of this thermodynamic transition? |
| </ol> | | </ol> |
| </li> | | </li> |
| Line 61: |
Line 37: |
|
| |
|
| <ol start="2"> | | <ol start="2"> |
| <li> <em> Entropy.</em> What happens to the entropy of the model when the critical temperature is reached, and in the low temperature phase? What does this imply for the partition function <math> Z</math>?</li> | | <li><em> Fluctuations, and back to average vs typical.</em> Similarly to what we did for the entropy, one can define an annealed free energy <math> f_{\text{a}}(\beta) </math> from <math> \overline{Z_N(\beta)}=e^{- N \beta f_{\text{a}}(\beta) + o(N)} </math>: show that in the whole low-temperature phase this is smaller than the quenched free energy obtained above. Putting all the results together, justify why the average of the partition function in the low-T phase is "dominated by rare events". |
| </ol>
| | </ol></li> |
| <br> | | <br> |
|
| |
|
| <ol start="3"> | | <ol start="3"> |
| <li><em> Fluctuations, and back to average vs typical.</em> Similarly to what we did for the entropy, one can define an annealed free energy <math> f^A </math> from <math> \overline{Z}=e^{- N \beta f^A + o(N)} </math>: show that in the whole low-temperature phase this is smaller than the quenched free energy obtained above. Putting all the results together, justify why the average of the partition function in the low-T phase is "dominated by rare events". | | <li> <em> Entropy crisis.</em> What happens to the entropy of the model when the critical temperature is reached, and in the low temperature phase? What does this imply for the partition function <math> Z_N</math>?</li> |
| </ol></li> | | </ol> |
| | <br> |
|
| |
|
| | <ol start="4"><li><em> Overlap distribution and glassiness.</em> |
| | Justify why in the REM the overlap when <math> N \to \infty</math> typically can take only values zero and one, leading to |
| | <center> |
| | <math> |
| | P_\beta(q)= \lim_{N \to \infty} \overline{P_{N, \beta}(q)}= \overline{I_2} \, \delta(q-1)+ (1-\overline{I_2}) \, \delta(q), \quad \quad I_2= \lim_{N \to \infty} \frac{\sum_\alpha z_\alpha^2}{\left(\sum_\alpha z_\alpha\right)^2}, \quad \quad z_\alpha=e^{-\beta E_\alpha} |
| | </math> |
| | </center> |
| | Why <math> I_2 </math> can be interpreted as a probability? Show that |
| | <center> |
| | <math> |
| | \overline{I_2} |
| | =\begin{cases} |
| | 0 \quad &\text{if} \quad T>T_f\\ |
| | 1-\frac{T}{T_f} \quad &\text{if} \quad T \leq T_f |
| | \end{cases} |
| | </math> |
| | </center> |
| | and interpret the result. In particular, why is this consistent with the entropy crisis? <br> |
| | <em> Hint: </em> For <math> T<T_f </math>, use that <math> \overline{I_2}=\frac{T_f}{T} \frac{d}{d\mu}\log \int_{0}^\infty (1-e^{-u -\mu u^2})u^{-\frac{T}{T_f}-1} du\Big|_{\mu=0}</math></li> </ol> |
| | <br> |
|
| |
|
| '''Comment:''' the low-T phase of the REM is a frozen phase, characterized by the fact that the free energy is temperature independent, and that the typical value of the partition function is very different from the average value. In fact, the low-T phase is also <math> a glass phase </math> in the sense discussed in the lecture. It is characterized by the fact that Replica Symmetry is broken, as one sees explicitly by re-deriving the free energy through the replica method. We go back to this in the next lectures/TDs.
| | == Comments== |
|
| |
|
| |
|
| === Problem 3: freezing as a localization/condensation transition ===
| | [[File:Suscept-experiment.png|thumb|left|x140px| Experimental measurements of the magnetic Field Cooled (top) and Zero-Field Cooled (bottom) susceptibility in a CuMn spin glass. Figure taken from C. Djurberg, K. Jonason, P. Nordblad, https://arxiv.org/abs/cond-mat/9810314]] |
|
| |
|
| In this final exercise, we show how the freezing transition can be understood in terms of extreme valued statistics (discussed in the lecture) and localization. We consider the energies of the configurations and define <math> E_\alpha= - N \sqrt{\log 2} + \delta E_\alpha </math>, so that
| | <ol> |
| <center><math>
| | <li><em> Glassiness.</em> The low-T phase of the REM is a frozen phase, characterized by the fact that the free energy is temperature independent, and that the typical value of the partition function is very different from the average value. In fact, the low-T phase is also <em> a glass phase </em> with <math> q_{EA}=1</math>. This is also a phase where a peculiar symmetry, the so called replica symmetry, is broken. We go back to this concepts in the next sets of problems. |
| {Z} = e^{ \beta N \sqrt{\log 2} }\sum_{\alpha=1}^{2^N} e^{-\beta \delta E_\alpha}= e^{ \beta N \sqrt{\log 2} }\sum_{\alpha=1}^{2^N} z_\alpha | |
| </math></center>
| |
| We show that <math> Z </math> is a sum of random variables that become heavy tailed for <math> T < T_c </math>, implying that the central limit theorem is violated and this sum is dominated by few terms, the largest ones. This can be interpreted as the occurrence of localization.
| |
|
| |
|
| | | <br> |
| <ol> | |
| <li> <em> Heavy tails and concentration.</em> Compute the distribution of the variables <math> \delta E_\alpha </math> and show that for <math> (\delta E)^2/N \ll 1 </math> this is an exponential. Using this, compute the distribution of the <math> z_\alpha </math> and show that it is a power law,
| |
| <center><math>
| |
| p(z)= \frac{c}{z^{1+\mu}} \quad \quad \mu= \frac{2 \sqrt{\log 2}}{\beta}
| |
| </math></center>
| |
| When <math> T < T_c </math>, one has <math> \mu<1 </math>: the distribution of <math> z </math> becomes heavy tailed. What does this imply for the sum <math> Z </math>? Is this consistent with the behaviour of the partition function and of the entropy discussed in Problem 2? Why can one talk about a localization or condensation transition?</li>
| |
| </ol>
| |
| <br> | | <br> |
|
| |
|
| <ol start="2">
| | <li><em> The physics: susceptibility and experiments.</em> The magnetic susceptibility of the REM can be computed adding a field to the energy function: <math> E(\vec{\sigma}^\alpha) \to E_\alpha + H \sum_i \sigma^\alpha_i</math>. Redoing the thermodynamics calculation in presence of the field (do its!!) one can show that in the REM |
| <li><em> Inverse participation ratio.</em> The low temperature behaviour of the partition function an be characterized in terms of a standard measure of localization (or condensation), the Inverse Participation Ratio (IPR) defined as: | |
| <center><math> | | <center><math> |
| IPR= \frac{\sum_{\alpha=1}^{2^N} z_\alpha^2}{[\sum_{\alpha=1}^{2^N} z_\alpha]^2}= \sum_{\alpha=1}^{2^N} \omega_\alpha^2 \quad \quad \omega_\alpha=\frac{ z_\alpha}{\sum_{\alpha=1}^{2^N} z_\alpha}.
| | \chi(\beta) = \frac{\partial m(\beta, H) }{\partial H}\Big|_{H=0}= |
| | \begin{cases} |
| | &\frac{\partial \tanh(\beta H) }{\partial H}\Big|_{H=0}= \beta \quad \text{if} \quad T \geq T_f\\ |
| | & \frac{\partial \tanh(\beta_f H) }{\partial H}\Big|_{H=0}= \beta_f \quad \text{if} \quad T <T_f |
| | \end{cases}. |
| </math></center> | | </math></center> |
| When <math> z </math> is power law distributed with exponent <math> \mu </math>, one can show (HOMEWORK!) that
| | where <math>m(\beta, H)</math> is the equilibrium magnetization. Therefore, the susceptibility does not diverge at the transition, as it happens in standard ferromagnets, but becomes flat (recall that what diverges at the spin-glass transition are non-linear susceptibilities, as discussed in Lecture 1 by Alberto). A similar behavior is found in measurements of the Field-Cooled susceptibility in spin glass systems, and it is captured by the Parisi's solution of the SK model with replicas, which therefore captures the physics probed in experiments. |
| <center><math>
| | |
| IPR= \frac{\Gamma(2-\mu)}{\Gamma(\mu) \Gamma(1-\mu)}.
| |
| </math></center>
| |
| Discuss how this quantity changes across the transition at <math> \mu=1 </math>, and how this fits with what you expect in general in a localized phase.
| |
| </li> | | </li> |
| </ol> | | </ol> |
| <br>
| | |
| | == Check out: key concepts == |
| | |
| | Freezing transition, entropy crisis, condensation, overlap distribution. |
| | |
| | == To know more == |
| | * Derrida. Random-energy model: limit of a family of disordered models [https://hal.science/hal-03285940v1/document] |
| | * Derrida. Random-energy model: An exactly solvable model of disordered systems [http://www.lps.ens.fr/~derrida/PAPIERS/1981/prb81.pdf] |
Goal: deriving the equilibrium phase diagram of the Random Energy Model (REM). Notion such as: freezing transition, entropy crisis, condensation, overlap distribution.
Techniques: saddle point approximation, thermodynamics, probability theory.
Problems
Problem 2: the REM: freezing transition, condensation & glassiness
In this Problem we compute the equilibrium phase diagram of the model, and in particular the quenched free energy density  which controls the scaling of the typical value of the partition function,
which controls the scaling of the typical value of the partition function,  . We show that the free energy equals to
. We show that the free energy equals to

At  a transition occurs, often called freezing transition: in the whole low-temperature phase, the free-energy is “frozen” at the value that it has at the critical temperature
a transition occurs, often called freezing transition: in the whole low-temperature phase, the free-energy is “frozen” at the value that it has at the critical temperature  .
.
We also characterize the overlap distribution of the model: the overlap between two configurations  is
is
 , and its distribution is
, and its distribution is

- The freezing transition.
The partition function the REM reads
 Using the behaviour of the typical value of
Using the behaviour of the typical value of  determined in Problem 1, derive the free energy of the model (hint: perform a saddle point calculation). What is the order of this thermodynamic transition?
determined in Problem 1, derive the free energy of the model (hint: perform a saddle point calculation). What is the order of this thermodynamic transition?
- Fluctuations, and back to average vs typical. Similarly to what we did for the entropy, one can define an annealed free energy
 from
from  : show that in the whole low-temperature phase this is smaller than the quenched free energy obtained above. Putting all the results together, justify why the average of the partition function in the low-T phase is "dominated by rare events".
: show that in the whole low-temperature phase this is smaller than the quenched free energy obtained above. Putting all the results together, justify why the average of the partition function in the low-T phase is "dominated by rare events".
- Entropy crisis. What happens to the entropy of the model when the critical temperature is reached, and in the low temperature phase? What does this imply for the partition function
 ?
?
- Overlap distribution and glassiness.
Justify why in the REM the overlap when
 typically can take only values zero and one, leading to
typically can take only values zero and one, leading to

Why  can be interpreted as a probability? Show that
can be interpreted as a probability? Show that

and interpret the result. In particular, why is this consistent with the entropy crisis?
Hint: For  , use that
, use that 
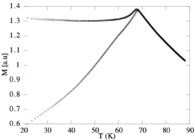
Experimental measurements of the magnetic Field Cooled (top) and Zero-Field Cooled (bottom) susceptibility in a CuMn spin glass. Figure taken from C. Djurberg, K. Jonason, P. Nordblad,
https://arxiv.org/abs/cond-mat/9810314
- Glassiness. The low-T phase of the REM is a frozen phase, characterized by the fact that the free energy is temperature independent, and that the typical value of the partition function is very different from the average value. In fact, the low-T phase is also a glass phase with
 . This is also a phase where a peculiar symmetry, the so called replica symmetry, is broken. We go back to this concepts in the next sets of problems.
. This is also a phase where a peculiar symmetry, the so called replica symmetry, is broken. We go back to this concepts in the next sets of problems.
- The physics: susceptibility and experiments. The magnetic susceptibility of the REM can be computed adding a field to the energy function:
 . Redoing the thermodynamics calculation in presence of the field (do its!!) one can show that in the REM
. Redoing the thermodynamics calculation in presence of the field (do its!!) one can show that in the REM

where  is the equilibrium magnetization. Therefore, the susceptibility does not diverge at the transition, as it happens in standard ferromagnets, but becomes flat (recall that what diverges at the spin-glass transition are non-linear susceptibilities, as discussed in Lecture 1 by Alberto). A similar behavior is found in measurements of the Field-Cooled susceptibility in spin glass systems, and it is captured by the Parisi's solution of the SK model with replicas, which therefore captures the physics probed in experiments.
is the equilibrium magnetization. Therefore, the susceptibility does not diverge at the transition, as it happens in standard ferromagnets, but becomes flat (recall that what diverges at the spin-glass transition are non-linear susceptibilities, as discussed in Lecture 1 by Alberto). A similar behavior is found in measurements of the Field-Cooled susceptibility in spin glass systems, and it is captured by the Parisi's solution of the SK model with replicas, which therefore captures the physics probed in experiments.
Check out: key concepts
Freezing transition, entropy crisis, condensation, overlap distribution.
To know more
- Derrida. Random-energy model: limit of a family of disordered models [1]
- Derrida. Random-energy model: An exactly solvable model of disordered systems [2]























