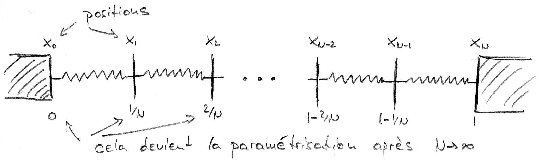T-II-2: Difference between revisions
Groupe 01 13 (talk | contribs) |
Groupe 01 13 (talk | contribs) |
||
| (14 intermediate revisions by the same user not shown) | |||
| Line 14: | Line 14: | ||
===Elasticity=== | ===Elasticity=== | ||
[[File:image009.jpg]] [[File:image008.jpg]] | |||
We write in two Step the elastic energy of a string with an elastic constant <math> k </math>. The shape of the string is defined by the function <math> y(x) </math>, where <math> 0<x <1</math> and | We write in two Step the elastic energy of a string with an elastic constant <math> k </math>. The shape of the string is defined by the function <math> y(x) </math>, where <math> 0<x <1</math> and | ||
<math> y(0)=y(1)=0</math>. | <math> y(0)=y(1)=0</math>. | ||
| Line 19: | Line 23: | ||
Step one: the discrete model | Step one: the discrete model | ||
* Write the elastic energy of a chain of N identical springs | * Write the elastic energy of a chain of N identical springs as a function of their position <math> y_1, y_2,\ldots,y_N </math> (See figure). | ||
* Determine the elastic constant of each spring in order to have | * Determine the elastic constant of each spring in order to have a string with an elastic constant <math> k </math> | ||
Step two: The continuum limit | Step two: The continuum limit | ||
| Line 27: | Line 31: | ||
===Gravity=== | ===Gravity=== | ||
We write now the gravitational potential as a functional of <math>y(x)</math>. We will use two different methods. We suppose that the total mass of the line in <math>M</math>. | We write now the gravitational potential as a functional of <math>y(x)</math>. We will use two different methods. We suppose that the total mass of the line in <math>M</math>. | ||
* Suppose that springs are massless and <math>N</math> identical masses are located at positions <math>y_1,y_2,\ldots</math> | * Suppose that springs are massless and <math>N</math> identical masses are located at positions <math>y_1,y_2,\ldots</math> | ||
| Line 50: | Line 45: | ||
<center><math> \int_{t_1}^{t_2} d t \int_0^1 d x \, \mathcal{L} (x,t) </math></center> | <center><math> \int_{t_1}^{t_2} d t \int_0^1 d x \, \mathcal{L} (x,t) </math></center> | ||
where <math> {\mathcal L}(x,t) </math> is the Lagrangian denisty | where <math> {\mathcal L}(x,t) </math> is the Lagrangian denisty | ||
* Write explicitly <math> {\mathcal L}(x,t) </math> | * Write explicitly <math> {\mathcal L}(x,t) </math> . | ||
* Write the equation of motion of the chain using the minimum action principle. This equation is the D'Almbert equation. | * Write the equation of motion of the chain using the minimum action principle. This equation is the D'Almbert equation. | ||
| Line 69: | Line 64: | ||
As a consequence of these fluctuations, the volume which contains a given mass <math>M </math> expands from the initial value <math>V_0 </math> to a new value <math>V_0 +\delta V</math>. The pressure inside the domain also fluctuates <math>P=P_0+\ldots </math>. The variation of potential energy is given by the work associated to the volume expansion: | As a consequence of these fluctuations, the volume which contains a given mass <math>M </math> expands from the initial value <math>V_0 </math> to a new value <math>V_0 +\delta V</math>. The pressure inside the domain also fluctuates <math>P=P_0+\ldots </math>. The variation of potential energy is given by the work associated to the volume expansion: | ||
<center><math> - \int_{V_0}^{V_0+\delta V} \, P\, d \vec{r}=-P_0 \delta V- \frac{1}{2} \left( \frac{\partial P }{\partial V}\right)_0 \delta V^2+\ldots </math> </center> | <center><math>V_0\, {\mathcal{V}(\vec r,t)} - \int_{V_0}^{V_0+\delta V} \, P\, d \vec{r}=-P_0 \delta V- \frac{1}{2} \left( \frac{\partial P }{\partial V}\right)_0 \delta V^2+\ldots </math> </center> | ||
where <math>\mathcal V(\vec r,t)</math>is the density of potential energy. | |||
Generally speaking, a sound wave in an ideal gas oscillates sufficiently rapidly that heat is unable to flow fast enough to smooth out any temperature perturbations generated by the wave. Under these circumstances, the gas obeys the adiabatic gas law, | Generally speaking, a sound wave in an ideal gas oscillates sufficiently rapidly that heat is unable to flow fast enough to smooth out any temperature perturbations generated by the wave. Under these circumstances, the gas obeys the adiabatic gas law, | ||
<center><math>P V^\gamma= </math> constant</center> | <center><math>P V^\gamma= </math> constant</center> | ||
where <math>\gamma</math> the ratio of specific heats (i.e., the ratio of the gas's specific heat at constant pressure to its specific heat at constant volume). This ratio is approximately <math>1.4 </math> for ordinary air. | where <math>\gamma</math> the ratio of specific heats (i.e., the ratio of the gas's specific heat at constant pressure to its specific heat at constant volume). This ratio is approximately <math>1.4 </math> for ordinary air. | ||
* Show that the total potential energy writes | * Show that the total potential energy, <math>W=\int \, d \vec{r} \, \mathcal V(\vec r,t)</math> writes | ||
<center><math> W= P_0 \int \, d \vec{r} \, \left( \sigma + \frac{\gamma}{2} \sigma^2\right) </math> </center> | <center><math> W= P_0 \int \, d \vec{r} \, \left( \sigma + \frac{\gamma}{2} \sigma^2\right) </math> </center> | ||
* Using the continuity equation of the gas show that <math> \sigma(\vec{r},t)= - \vec{\nabla} \cdot \vec{u}(\vec{r},t) </math> and write the potential energy as a function of <math> \vec{u}(\vec{r},t) </math> | * Using the continuity equation of the gas, <math>\partial_t \rho=-\rho_0 \vec{\nabla} \cdot \partial_t \vec{u}(\vec{r},t)</math>, show that <math> \sigma(\vec{r},t)= - \vec{\nabla} \cdot \vec{u}(\vec{r},t) </math> and write the potential energy as a function of <math> \vec{u}(\vec{r},t) </math> | ||
===Lagrangian description=== | ===Lagrangian description=== | ||
* Show that the Lagrangian density writes | * Show that the Lagrangian density writes | ||
<center><math> \mathcal{L} (\vec{r},t) = \frac{\rho_0}{2} \dot{\vec{u}}^2 + | <center><math> \mathcal{L} (\vec{r},t) = \frac{\rho_0}{2} \dot{\vec{u}}^2 + P_0 \vec{\nabla} \cdot \vec{u} -\frac{\gamma}{2} P_0 (\vec{\nabla} \cdot \vec{u})^2 </math></center> | ||
* Show that the associated equation of motion | * Show that the associated equation of motion | ||
<center><math> \rho_0 \frac{\partial^2 \vec{u}}{\partial t^2} -\gamma P_0 \vec{\nabla} \vec{\nabla} \cdot \vec{u}=0 </math></center> | <center><math> \rho_0 \frac{\partial^2 \vec{u}}{\partial t^2} -\gamma P_0 \vec{\nabla} \vec{\nabla} \cdot \vec{u}=0 </math></center> | ||
* Using the continuity relation <math> \sigma= - \vec{\nabla} \cdot \vec{u} </math> show that the latter equation corresponds to the D'Alembert Equation for the scalar field <math> \sigma </math> | * Using the continuity relation <math> \sigma= - \vec{\nabla} \cdot \vec{u} </math> show that the latter equation corresponds to the D'Alembert Equation for the scalar field <math> \sigma </math> | ||
This scalar | This scalar field behaves exaclty like an elastic scalar field. | ||
* Determine the sound velocity. | * Determine the sound velocity. | ||
Latest revision as of 16:49, 15 November 2011
Variational methods
Elastic line
During the class you determined the shape of an non-extensible string in a gravitational potential. Here, we study a different situation: the case of an elastic line. Hooke's law states that Elastic energy is proportional to the square of the global elongation.
Elasticity
We write in two Step the elastic energy of a string with an elastic constant . The shape of the string is defined by the function , where and .
Step one: the discrete model
- Write the elastic energy of a chain of N identical springs as a function of their position (See figure).
- Determine the elastic constant of each spring in order to have a string with an elastic constant
Step two: The continuum limit
- Take the limit and express the elastic energy as a functional of . (Input: use and )
Gravity
We write now the gravitational potential as a functional of . We will use two different methods. We suppose that the total mass of the line in .
- Suppose that springs are massless and identical masses are located at positions
- Combine your finding for elasticity and gravity and show that the shape of the elastic line is parabolic
Dynamics
We want to study the motion of the elastic line in absence of gravity and in presence of small oscillations. The shape of the line is now time dependent .
- Write the kinetic energy associated to the masses located at . Take the continuum limit.
The action associated to the elastic chain is
where is the Lagrangian denisty
- Write explicitly .
- Write the equation of motion of the chain using the minimum action principle. This equation is the D'Almbert equation.
Sound waves in ideal gas
Consider a uniform ideal gas of equilibrium mass density and equilibrium pressure . Let us investigate the longitudinal oscillations of such a gas. Of course, these oscillations are usually referred to as sound waves.
Kinetic Energy
In a first time we can start from the discrete description of a system of N atoms at positions .
- Write the kinetic energy, , for this system as a function of mass of the atom .
- Consider the limit of small oscillations. Take the continuum limit: and show that the kinetic energy writes
Potential Energy
In order to write the full Lagrangian we need to find the potential energy associated to the oscillations. During the oscillations the mass density can have small fluctuations
The scalar field represents the relative fluctuation of the density.
As a consequence of these fluctuations, the volume which contains a given mass expands from the initial value to a new value . The pressure inside the domain also fluctuates . The variation of potential energy is given by the work associated to the volume expansion:
where is the density of potential energy.
Generally speaking, a sound wave in an ideal gas oscillates sufficiently rapidly that heat is unable to flow fast enough to smooth out any temperature perturbations generated by the wave. Under these circumstances, the gas obeys the adiabatic gas law,
where the ratio of specific heats (i.e., the ratio of the gas's specific heat at constant pressure to its specific heat at constant volume). This ratio is approximately for ordinary air.
- Show that the total potential energy, writes
- Using the continuity equation of the gas, , show that and write the potential energy as a function of
Lagrangian description
- Show that the Lagrangian density writes
- Show that the associated equation of motion
- Using the continuity relation show that the latter equation corresponds to the D'Alembert Equation for the scalar field
This scalar field behaves exaclty like an elastic scalar field.
- Determine the sound velocity.